One of the most common corrosion problems you will be asked to solve is how a tank, open to the atmosphere, corrodes and how you can control the rate of corrosion by simply covering the tank and regulating the pressure. Here is what is usually given:
T = 25oC
P = 1 atm
Corrosion Rate = 2.4 g Fe/cm2-yr
Say that O2 reduction is limited by mass transfer of dissolved O2 to the tank wall and the solubility of dissolved oxygen can be related to its partial pressure:
CO2 = 1.28x10-3 PO2
The partial pressure is in atm and the concentration is in mol/L. The oxidation of Feo is kinetically controlled in the Tafel region:
iO = 0.8x10-8 amp/cm2
Tafel Slope = 1/120 mV-1
ne = 1
Acceptable Corrosion Rate = 0.01 g Fe/cm2-yr
CFe2+ = 10-6 mol/L at all times
Feo -> Fe2+ + 2e-, -0.440 VSHE
And you are asked to determine the total pressure the headspace should be evacuated to to attain the acceptable corrosion rate.
Solution:
You are given that the O2 reduction is mass transfer limited so right away you can modify your general equation from:
To:
From the given information you can find the concentration of dissolved oxygen in the bulk as well as the current from the Faraday’s law. Please see my earlier posts for more information on how to use Faraday’s to calculate current given a deposition rate.
From the given relationship between pressure and concentration, you can determine the bulk concentration of dissolved oxygen:
Now that you have all the required information, you can calculate the km value.
The next step is to determine the current density at the acceptable corrosion rate using the same line calculation as before:
Looking at the mass transfer limitation equation, we only have 1 unknown – the bulk concentration of dissolved oxygen, which is calculated via:
Great, now we can use the given relationship for partial pressure of oxygen and concentration to determine the partial pressure of oxygen in the headspace:
This is not the end, because oxygen only accounts for 21% of the composition of air, so the total headspace pressure has to be:
I apologize how bad some of the equations look. I really have to look into finding a new blog editor. If this has helped you in any way, please click on some of the ads.
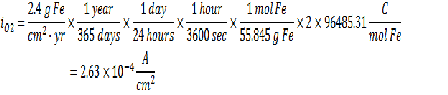
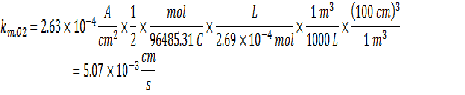

0 comments:
Post a Comment